Eurythmy as Inexhaustible Complex of Tensions
Let us recall first the nature and the role of the chorus. The χορός (khorόs) was a homogeneous, non-individualized group of performers, who commented with a collective voice on the dramatic action. It consisted of between 12 and 50 players, who variously danced, sang or spoke their lines in unison and sometimes wore masks. Important is to note that choruses existed in the context of tragedy, comedy, and also in satyr plays, which were all performed at least in the 6th and 5th centuries during Dionysian celebrations. In the more ancient rural Dionysia, after the procession, there were contests of dancing and singing, and choruses led by a χορηγός (khorêgόs) would perform dithyrambs, sing hymns and dance in honor of Dionysus. Some ot these rural festivals may have included dramatic performances, possibly of the tragedies and comedies that had been produced at the City Dionysia the previous year. In the City Dionysia, after the procession, the khorêgoí led their choruses in the dithyrambic competitions. These were extremely competitive, and the best flute players and celebrity poets offered their musical and lyrical services. During the 5th century BC, five days of the festival were set aside for performance of three tragedies and one satyr play. The other two days were likely devoted to dithyrambic contests until 487/6 BC when comic poets were officially admitted to the agons and eligible for their own prizes.
Nietzsche pays a lot of attention to these half religious half theatrical practices. We remember that, according to him, in the chorus performances the musical rhythm followed the irregular rhythm of poetry and both followed in turn the ever changing rhythm of the dance.
Principles: intimate fusion of words and music, but in a way that the duration of the spoken word generally prevails. Infinite mimicry [Mimik]: the music has no absolute character. Insofar as it is imitative, it has no regular measure [Taktgleichheit]. (at least [it is] not necessary) (Griechische Rhythmik, KGA II3, p. 192, my trans.)
In the same way as in The Dionysiac World View, Nietzsche describes the chorus dance “which was not a whirling dance” as “a nice going.” But the last sentence introduces a new parameter: this “nice going [...] naturally [met] with uneven measures.”
An important law [was] that the measure [Takt] originally was part of the orchestics: the singer would adjust to the dance (which was not a whirling dance [kein Wirbeltanz], but a nice going [sondern ein schönes Gehen]) Naturally meeting with uneven measures [ungliechem Takte] multiple κινήσεις [kinêseis – movements] of the dancers. (Zur Theorie der quantitirenden Rhythmik, 1870-1872, KGA II3, p. 270, my trans.)
The primacy of dance upon poetry and music probably explains that unlike the Moderns, the Greeks used a quite larger number of measures [1]. Besides the four simple measures which were identical to modern ones (3/8, 3/4, 2/4, 6/8), there existed a dozen others (4/8, 5/8, 9/8, 4/4, 5/4 or 10/8, 12/8, 15/8, 16/8, 18/8, 20/8, 25/8) (Griechische Rhythmik, KGA II3, p. 107-109). This rhythmic wealth resulted in frequent use of uneven five-beat measures (5/8, 5/4, 15/8, 25/8) which survived in modern Europe “here and there in Folksong” but were outlawed in art music. A ternary measure could be even associated with a quinary measure which formed a particularly unbalanced meter: the dochmiach, expressing in tragedy extreme agitation or distress.
We [Moderns] have 2 kinds of measure [Taktarten], even or uneven [gerade u. ungerade]. In the Middle Ages the two-part [measure] was called according to Platonic symbolism genus imperfectum, the three-part [measure] genus perfectum. A third kind of measure [Taktart] the five-part [measure] appears here and there in Folksong. It was by no means coordinated [with the previous ones]. But it was [coordinated] since the 6th century (in ὑπόσχημα [hupóskhêma] and chorus songs of the comedy, then with the three-part mixt in the μονῳδίαι [monôidíai] of the Tragedy). (Griechische Rhythmik, KGA II3, p. 108, my trans.)
Such mixts resulted in uneven poetic meters, since two heterogeneous kinds of measure were then brought together. Nietzsche notices for instance the blending of a 5/4 measure with a 2/4 measure in a piece, the nome in honor of Athena, composed by Olympos who according to legend was originally from Phrygia.This rhythmic disproportion, probably invented by performers-poets-musicians from Asia Minor, was therefore one of the first forms that the symbiosis of Dionysian and Apollonian principle took in Greek art.
When studying Aristoxenus and Aristides Quintilianus, Nietzsche notes that pόdes álogoi (irrational feet) were the “most striking features in Greek rhythmic” (Griechische Rhythmik, KGA II3, p. 114). But the meaning of this classification is not completely clear. As we have already seen (vol. 1, chap. 2), the term álogon appears in Plato’s Timaeus. Alogía, i.e. want of reason, unreasonable conduct, absurdity, characterizes the initial chaos before its shaping and organization by the Demiurge.
[...] that burdensome mass which afterwards adhered to him of fire and water and earth and air, a mass tumultuous and irrational [θορυβώδη καὶ ἄλογον ὄντα – thorubôdê kaì álogon ónta] [...] (Timaeus, 42d, trans. W.R.M. Lamb)
This chaos in its “natural condition” is “alόgôs kai ámetrôs” which means irrational and without measure, immense, excessive, boundless. Then the Demiurge shapes and organizes it “by means of forms and numbers.”
Before that time, in truth, all these things were in a state devoid of reason or measure [πάντα ταῦτ᾽ εἶχεν ἀλόγως καὶ ἀμέτρως – pánta taũt’ eikhen alógôs kaì amétrôs] but when the work of setting in order this Universe was being undertaken, fire and water and earth and air, although possessing some traces of their own nature, were yet so disposed as everything is likely to be in the absence of God; and inasmuch as this was then their natural condition, God began by first marking them out into shapes by means of forms and numbers [διεσχηματίσατο εἴδεσί τε καὶ ἀριθμοῖς – dieskhêmatísato eídesí te καὶ arithmoĩs]. (Timaeus, 53a-b, trans. W.R.M. Lamb)
Drawing on this view, Timaeus contrasts the “irrational pleasure” felt by the crowd with the real “intellectual pleasure” which grows in our soul from harmony and rhythm. Both harmony and rhythm are “auxiliary to the inner revolution of the Soul” to help it achieve or recover balance.
Concerning sound also and hearing, once more we make the same declaration, that they were bestowed by the Gods with the same object and for the same reasons; for it was for these same purposes that speech was ordained, and it makes the greatest contribution thereto; music too, in so far as it uses audible sound, was bestowed for the sake of harmony. And harmony, which has motions akin to the revolutions of the Soul [τῆς ψυχῆς περιόδοις – tês psukhês periódois] within us, was given by the Muses to him who makes intelligent use of the Muses, not as an aid to irrational pleasure [ἡδονὴν ἄλογον – hêdonên álogon], as is now supposed, but as an auxiliary to the inner revolution of the Soul, when it has lost its harmony, [ἀνάρμοστον ψυχῆς περίοδον – anármoston psukhês períodon] to assist in restoring it to order and concord with itself. And because of the [ametrical/irregular/erratic] [ἄμετρον – ámetron] condition, deficient in grace, which exists in most of us, rhythm [ῥυθμός – rhuthmós] also was bestowed upon us to be our helper by the same deities and for the same ends. (Timaeus, 47c-e, trans. W.R.M. Lamb, my mod.)
Harmony and rhythm are gifts of the gods which aim at infusing measure and grace into men in order to help them to overcome the original alogía and to get in tune with the divine geometrical and arithmetical harmony.
Nietzsche knows about this but he notices with great interest that both Aristoxenus and Aristides Quintilianus transformed this Platonic cosmological concept into a rhythmic category. Some Greek meters were composed of two conflicting feet, or in some particular line a heterogeneous meter broke the rhythmic continuity. These phenomena would be named alogía “irrationality,” because arithmetical proportions of the meter or the line were affected. However none of them considered them as chaotic, abnormal, or even distasteful. It is as if alogía would not denote any longer, in a precise Platonic way, something belonging to the “tumultuous mass” before God’s shaping of the world but to things belonging to the new world born thanks to his rhythmic intervention. Or, if it would be, in a more Aristotelian way, a mere technical category necessary to describe the complexity of Greek music as these thinkers perceive it.
In his partly preserved Elements of Rhythmic, II, 20, Aristoxenus (4th cent. BC) mentions the choree or choriamb (— u u —) as an ἄλογος – álogos meter which is composed of a trochee (— u) followed by an iamb (u —), in other words of two feet whose arsis and thesis are exactly opposed. Similarly, in his famous treaty On Music I, 14, Aristides Quintilianus (2nd or 3rd century AD) emphasizes that all álogoi meters can be analyzed and that there are never entirely devoid of any order.
Nietzsche in turn traces the alogía to the association, in the same meter, of a trochee with an iamb (— u u —), or a dactyl with an anapest (— u u u u —) (Griechische Rhythmik, KGA II3, p. 177). He dedicates several pages to the analysis of the various “irrational” meters, which he calls khoreîos (— u u u) and bakkhêîos (u — — u). He notices that irrationality is also produced by the introduction of a heterogeneous meter into a line of verse, for instance a dactyl (— u u) or a spondee (— —) in the middle of a iambic trimeter (ibid., p. 181-187).
All these configurations are disturbing to the Moderns because the long syllables—or for us the stressed syllables—are either opposing or facing each other. In French prosody, for instance, although Meschonnic has shown their common existence in poetry as soon as the middle of the 19th century, as well as in ordinary language, this kind of rhythmic configuration called contre-accent was considered as cacophonous and banned by metricians and purists until late in the 20th century (Dessons & Meschonnic, 1998, p. 152-156). Likewise such plays with long and short syllables had in Greece an expressive function and was an important part of the effect produced on the listener by the rhythm of a poem or a piece of theater.
Strikingly, in order to give a modern account of that kind of phenomenon, Nietzsche imports into rhythmic the concept of “dissonance” which traditionally pertains to harmony. Throughout the 19th century metricians have been discussing the “logaoedic verse,” a notion that is now unfortunately considered as obsolete. He claims that this kind of verse, which unevenly blends equal metrical feet (anapest, dactyl) with double feet (iamb, trochee), proves the existence in ancient Greek poetry of a specific “dissonant” Dionysian “time-measure” which was complex and irrational, free from any architectural symmetry and featuring in most expressive dances.
I believe that the impulse to play with strong dissonances of time-measure [mit starken Dissonanzen des Zeitmaaßes] is a fruit of the Dionysus cult. The logaoedic verses are therefore not to be used with pauses on the same measures [zu gleichen Takten]. They are characterized by the change of measure [Taktwechsel] (which is strongly mimic). (Rhythmische Untersuchungen, KGA II3, p. 329, my trans.)
The name βακχεῖοι given to certain kinds of verse with uneven beat number seems to him a good piece of evidence of this origin since Βακχεία meant feast of Bacchus, Bacchic frenzy, or revelry.
Previously βακχεῖοι in the Dionysian and Demeter cult songs. (Griechische Rhythmik, KGA II3, p. 113, my trans.)
The notion of “rhythmic dissonance” acquires little by little a greater importance in Nietzsche’s reflection. To elaborate it, he first relies on Aristoxenus and Böckh to raise the problem of the spondee duration within a iambic trimeter.
The light [leichte] part of the measure is lengthened by a little. Aristoxenus reports the occurrence of such measures. The irrational τρίσημοι [trísêmoi], as Boeckh has recognized, are identical with the spondee, which appear in the iambic meters in the uneven [places] and in the trochaic in the even places, instead of the iamb and trochee. E.g. trimeter
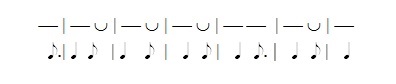
(Griechische Rhythmik, KGA II3, p. 114, my trans.)
The initial long and the second long of the spondee in the fourth foot are transcribed as dotted eighth note. Two long syllables may thus have different durations. Similarly, the duration of a long syllable is not always equivalent to that of two shorts.
— is not quite as long as u u
(Griechische Rhythmik, KGA II3, p. 195, my trans.)
Then Nietzsche generalizes his finding. “Rhythmic dissonance” was commonplace in Greek poetry.
Important that in the main meters in hexameter and iamb the ἀλογία [alogía – irrational] had an important place.
Perhaps the following is to be distinguished in the trimeter:
— u u and u u — real ἀλογίαι [alogíai – irrationals]
— — rational measure.
Thus the dissonance with — — was stronger than with — u u and u u — (Rhythmische Untersuchungen, KGA II3, p. 337, my trans.)
According to this rule, an irrational spondee (— —) has not always exactly the same duration as a dactyl (u — —) or an anapest (u u —). Hence the substitution of two short syllables with a long one modifies the temporal proportions within the dactylic hexameter or the iambic trimeter. Nietzsche’s conclusion is that this phenomenon introduces in Greek poetry “a lot of fine [rhythmic] dissonances.”
Thus, we see that both first and second places of the 1, 3, 5 feet can be irrational. — — must be understood either as α— or —α. This results in a lot of fine dissonances. (Rhythmische Untersuchungen, KGA II3, p. 337, my trans.)
The question naturally arises as how much this kind of “irrationality” changes the tempo. In ancient Greek tempo is called ἀγωγή – agôgê. But Nietzsche remarks that this term actually denotes a difference κατά μέγεθος – katá mégethos, (II, 22) viz. according to the magnitude or the loudness of sound.
ἀγωγή does not mean tempo in Aristoxenus. It is the διαφορά κατά μέγεθος. (Griechische Rhythmik, KGA II3, p. 146, my trans.)
One cannot therefore assimilate the alogía to a modification of the pulsation: the introduction of irrational short and long syllables does not induce any change in tempo as in 19th century music. Acceleration is not here a relevant category and is substituted with a subtle play between syllable durations modified by the process of alogía.
Acceleration is expressed only by long and short [syllables]. A tempo difference did not exist. (Griechische Rhythmik, KGA II3, p. 146, my trans.)
At the very beginning of Grieschische Rhythmik, Nietzsche notices that Aristoxenus thought of rhythm in an opposite way to the Moderns, viz. from the khrónoi prôtoi to the measure and from the measures to the whole of rhythm, and not the other way around. This makes him a “rhythmician” and not a “metrician.”
In the terminology of the actual metricians πούς [poús – foot] is the word for “measure” [Takt], among the rhythmicians ῥυθμός [rhuthmόs – rhythm]. It is different with Aristoxenus. The rhythm is here the whole of a series of measures. Not merely βάσις [básis] and ἄρσις [ársis] but also the ὅλος πούς [hόlos poús – entire foot] is called by Aristoxenus χρόνος ποδικός [khrόnos podikόs – time of a metrical foot]. (Griechische Rhythmik, KGA II3, p. 103, my trans.)
But this inversion between primary and secondary elements entailed also that the rhythm was not regular. Indeed, each measure (“secondary element”) was composed of “primary elements” which were not of equivalent durations. The khrόnos prôtos had “no absolute time-length.”
We determine the measure range according to eighth, quarter, or half notes. We distinguish between 6/8 and 3/4 measures, 6/4 and 3/2 (i.e. at the same time κατὰ γένος [katà génos]. The Ancients have it differently. They determine the smallest measure unit [Maaßeinheit] χρόνος πρῶτος [khrόnos prôtos – first time unit]. The measure of 2, 3, etc. χρόνοι πρῶτοι [khrόnoi prôtoi] is called χρόνος δίσημος [khrόnos dísêmos – of two times], τρίσημος [trísêmos – of three times] : the χρόνος πρῶτος [khrόnos prôtos] [has] no absolute time-length, only by tempo. (Griechische Rhythmik, KGA II3, p. 107, my trans.)
In another fragment of Grieschische Rhythmik entitled “Tactwechsel und Tactgleichheit” –“Measure change and measure regularity,” Nietzsche emphasizes the variability of the agôgê in ancient Greek dance, poetry and music. Each piece had no fixed and regular tempo, rather each particular content determined the specific speed to be adopted. Thus poet-musicians could reconcile at will the regularity of the rational meters with the irregularity of the irrational meters.
The mathematical equality of measures was sought in the ἁπλοῖ ῥυθμοί [haploĩ rhuthmoí – simple rhythms]. In the others it was on the contrary (neglected) repealed [aufgehoben]. (Griechische Rhythmik, KGA II3, p. 171, my trans.)
Contrary to what modern metricians as Westphal or Rossbach asserted, the Greek performing arts were not subject to arithmetic nor based, as most 19th century thinkers believed, on symmetrical architecture. Nietzsche severely criticizes modern specialists who cut Greek lines of verse into equal measures following the rule of quadrature.
The trimeters are measured according to dipods. The metricians grasp the measures as whole and full four-timed measure. On this the ancient Greek had a finer judgment. (Rhythmische Untersuchungen, KGA II3, p. 334, my trans.)
This error entirely obliterates both subtle and powerful effects of Greek rhythmic, which the poet-musicians obtained thanks to the unevenness of the measures and the asymmetry of the periods. One cannot help but think about the argument against symmetry, evenness and regularity developed a few years later by Verlaine and the Symbolists.
In another fragment entitled “Tactgleichheit,” Nietzsche emphasizes the opposition between a narrow conception of rhythm, reduced to a regular, symmetrical and mathematical pendulum movement, and a broader conception which calls on the “psychological knowledge” of the interpreter and places great importance on irregularity and dissymmetry.
Mathematically, two measures [Takte] are never equal: the more spiritually the representation is apprehended, the more delicately individualized is the measure [Takt], first by its duration [Dauer] (ἀγωγή), then by its ictuses (by its declamation), and thirdly, by the duration [Dauer] of its individual parts. In lines and periods, this individuality now increases, the architectural stiffness is the death of performance. For this reason the conductor must not be a machine or a chronometer. The correct apprehension of the tempo of a subsequent piece of music is a psychological knowledge: the innermost essence of two successive pieces of music is expressed through the feeling produced by the differently chosen measures [Takte]. Just as the form of the leaf is always the same according to the idea, and in reality never the same, it is a similar situation with the equality of measures, periods, and strophes. The pendulum hits us painfully: it gives the mathematical skeleton. How will this be now stuffed with meat? (Aufzeichnungen zur Rhythmik und Metrik, KGA II3, p. 205, my trans.)
All evidence gathered by Nietzsche challenge the whole European classical and Vitruvian aesthetic tradition. The eurhythmic symbolization of the affects cannot result from due proportion and symmetry, i.e. from a Platonic rhythm which would only replicate an abstract and fixed Form—as if performing arts should conform to the rules of architecture. Rather, the arts regain their aesthetic autonomy, architecture is not considered a model anymore, and eurhythmy appears from “the feeling produced by the differently chosen measures,” that is from the organization of the performance, its particular ways of flowing. Greek poetry, far from being a rigid an regular structure, becomes a fluid medium which is to be shaped each time eurhythmically by the performer. It is much closer to the pre-Platonic Democritus’ and Xenophon’s rhuthmόs, than to its Platonic conception (see vol. 1).
As a matter of fact, in 1875 Nietzsche draws the logical conclusion from this reversal of perspective by transposing the rhythmic freedom of Greek performing arts into architecture. Actually, he claims, even the most rigid art of all is subject to that kind of fluid eurhythmy which is endowed with “animatedness instead of a mechanical movement.”
The unmathematical oscillation of the column in Paestum, for instance, is an analogue to the modification of the tempo: animatedness instead of a mechanical movement. (eKGWB/NF-1875,5[86] — Spring–Summer 1875, my trans.)
If we now look from the Notes on Rhythm back at The Birth of Tragedy, we realize that, in his early years, Nietzsche did not only assert the existence in ancient Geek art of two metaphysical principles, but that he philologically, linguistically and poetically showed how these two blended into one complex rhythmic.
Yet, I do not agree with Christophe Corbier, to whom I am indebted for a lot of these examples, when he claims at the end of his survey that eurhythmy is conceived by Nietzsche as a mere opposite to alogía, therefore composing with the latter a pair of principles analogous for performed poetry, music and dance, to those governing them at the metaphysical level.
From his study of Aristoxenian rhythm, Nietzsche deduces an essential rule of Hellenic art: Greek art, in its apogee, does not rest on an absolute regularity, on an infrangible symmetry, but on the indissoluble union of eurhythmy and its opposite, alogia, as indicated by a fragment of 1870-1871: “The genial sense of proportion, developed in the Greek language, and music and sculpture, is revealed in the moral law [Sittengestez] of the measure [des Maaßes]. The dionysian cult adds the ἀλογία to it.” (eKGWB/NF-1870,7[2] — End 1870-April 1871, my trans.) (Corbier, 2009)
In this fragment, Nietzsche does indeed allude to the Greek “genial sense of proportion” as revealed “in the moral law of the measure.” And he contrasts “Maaß – measure,” which in German as in English means quantity, dimension and moderation, with alogía thus implicitly referred to quality, modulation and excess. But this is a very early statement and a rather isolated one. It is, in my opinion, misleading to reduce it to a simplistic embitterment of a too sweet and gentle classicist aesthetics by a romantic drop of frenzy and excess.
I would rather think, based on the very pieces of evidence which Christophe Corbier helped to collect, that Nietzsche proposes an entirely new conception of eurhythmy which has nothing to do anymore with the Platonic conception, be it ancient or modern (see vol. 1, chap. 2 and vol. 2, chap. 3), and a lot with what began to come into view when we studied Diderot (vol 2. chap. 1), the German Romantics (chap. 2), and the greatest poets of the second half of the 19th century (chap. 5).
Whereas in classical—i.e. Platonic—art theories eurhythmy entailed due proportion, symmetry and fixity, i.e. a translation of heavenly perfection into the earthly world, Nietzsche describes it as produced by a wealth of intertwined measures, a succession of seemingly regular meters which are actually deeply affected by irregularities, contre-accents and rhythmic dissonances, moreover by a set of dynamic relations that are to be woven together each time anew during performance. Hence instead of a Platonic fixed eurhythmy, Nietzsche suggests a concept which is closer to what it probably was before Plato and which is also reminiscent of what Hölderlin had in mind when he tried to give an account of the dynamics of tragedy (see above chap. 2). It reflects an inexhaustible rhythmic complex of tensions that is available to any new performer, listener or viewer and that allows him/her to develop his/her performance, audition and vision each time in a new way, i.e. that entails an infinite meaning power deeply engrained in each work of art and therefore ensures a successful symbolization of the performers’ as much as the audience’s affects.

